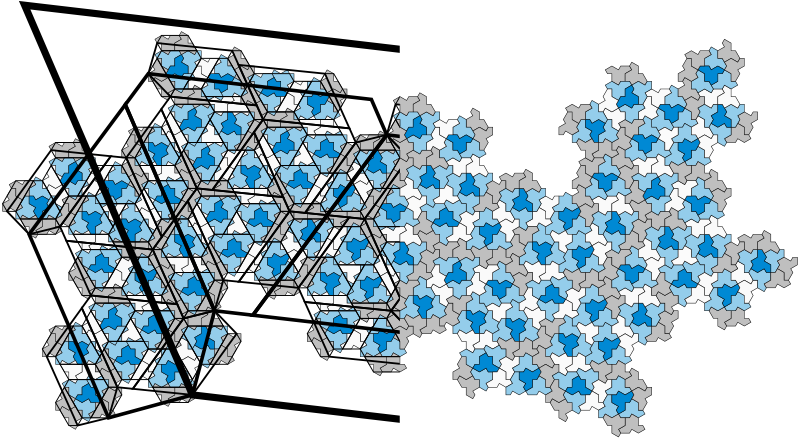
An aperiodic monotile

David Smith, Joseph Samuel Myers, Craig S. Kaplan, and Chaim Goodman-Strauss, 2023

An aperiodic monotile, sometimes called an "einstein", is a shape that tiles the plane, but never periodically. In this paper we present the first true aperiodic monotile, a shape that forces aperiodicity through geometry alone, with no additional constraints applied via matching conditions. We prove that this shape, a polykite that we call "the hat", must assemble into tilings based on a substitution system. The drawing above shows a patch of hats produced using a few rounds of substitution.

This page collects the resources associated with this work. We invite you to look at all of the following.

Tools and links

• A preprint of the paper is available on the arXiv.

• In May we published a follow-up paper, A chiral aperiodic monotile, in which we exhibit shapes that tile aperiodically without reflections. Please visit the page about that paper for more information.

• You can create your own patches of hats, and save them as PNG or SVG files, using an interactive application that runs in your web browser. You can also get your own copy of the source code (with a BSD 3-clause license). If you prefer to experiment with moving hats around and building patches manually, please see this excellent Mathigon polypad environment created by Dan Anderson.

• The hat is one member of a continuous family of shapes that are all aperiodic, and that all tile the plane in the same way. We have created an animation that moves smoothly through this family of shapes. You can watch it on YouTube, or download your own copy (which you can more easily watch looped).

• If you are interested in laser-cutting or 3D printing your own hats, there's a convenient collection of files giving hat outlines in various formats, assembled by Christian Lawson-Perfect.

• In the paper we give two different proofs of aperiodicity. One of them relies on a computer-assisted case-based analysis. For validation purposes, we re-implemented this analysis as a Python program. You can download the source code for this program, run it yourself, and check its correctness.

Sample images

Here are some sample images you can use in publications, media, etc. Feel free to modify these images to suit your tastes.

All images, and the MP4 animation above, are licensed under a Creative Commons Attribution 4.0 International License.

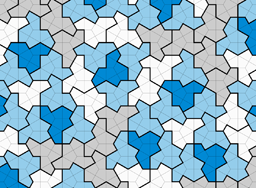
A zoomed-in patch of hat tiles, each one decorated with fine lines showing the underlying kites.
[1200x882 PNG] [Scalable PDF]

An alternative patch, more zoomed out and using a warmer palette.
[1200x755 PNG] [Scalable PDF]

A still more zoomed out patch with the same colouring as the first example, with a local centre of threefold rotation in the centre of the drawing.
[1200x1200 PNG] [Scalable PDF]

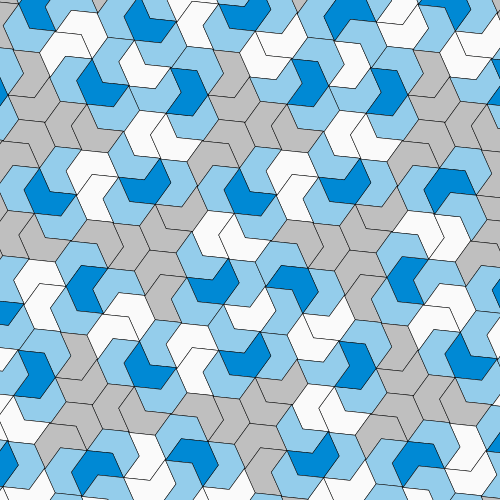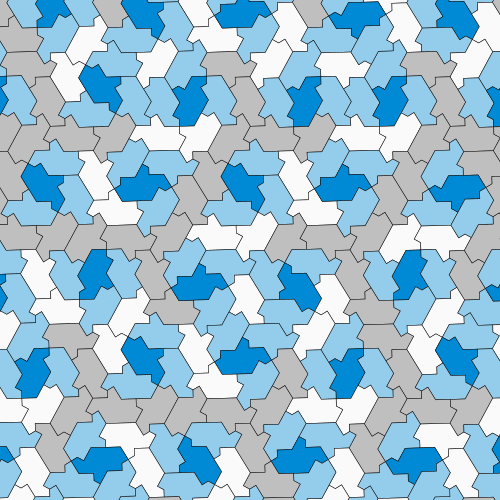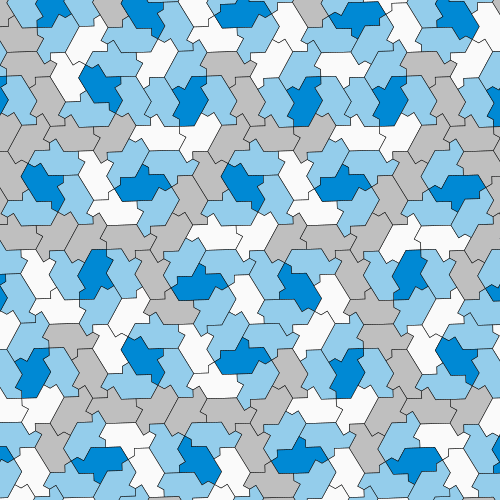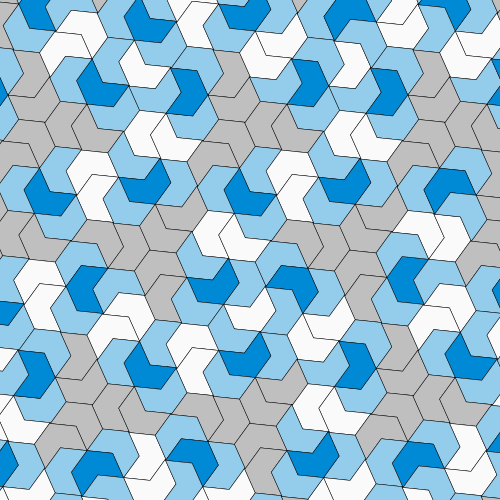
A looping animated GIF similar to the animation mentioned above. [500x500 GIF]

If you would like to contact us about this paper, please email me at csk@uwaterloo.ca.