Thesis page 35

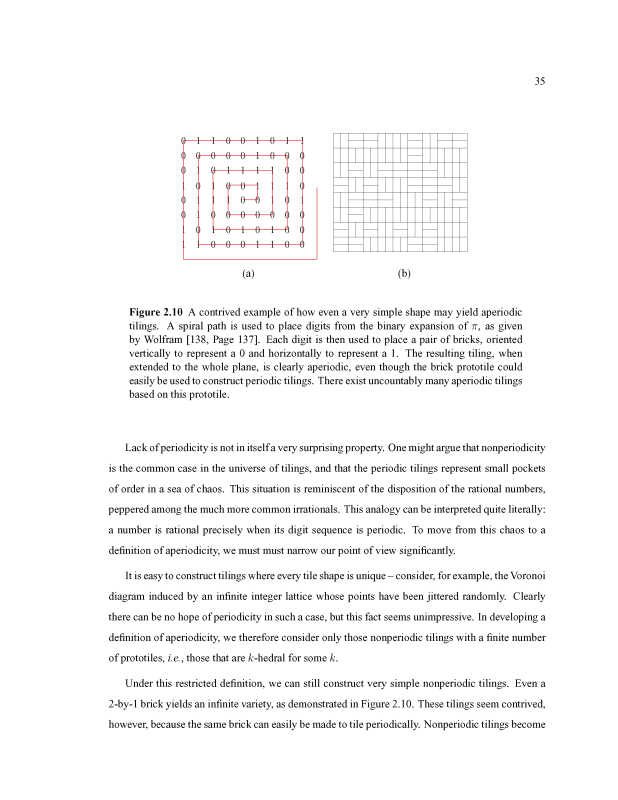
A few months before I wrote my dissertation, Stephen Wolfram's monumental A New Kind of Science came out. Now you can say whatever you like about the content of the book (I'll refrain from offering my opinion here). But in form, ANKOS is indisputably an object of great beauty. It's wonderfully laid out, dense with figures, and well written with a smooth division between readable exposition and technical details. I knew that somehow, I needed to include a reference to Wolfram from my work.

My opportunity came here, with a simple demonstration of a nonperiodic but not aperiodic tiling (reading the page again, I see that "nonperiodic" should be substituted for "aperiodic" in the figure caption). I knew that you could encode any real number between 0 and 1 using this spiral layout of tile pairs with each rotation storing a bit. Then I remembered that Wolfram had included a prefix of π in binary, and I had the chance I needed to cite him.

Interestingly, nobody who read my thesis pointed out a potentially serious problem with this figure. I boldly asserted that the resulting tiling of the plane is clearly not periodic. Actually, I never thought it was immediately clear, because of the funny spiral layout. Certainly there can be irrational numbers that yield periodic tilings when their binary expansions are depicted as tilings in this manner. So is π sufficiently ill-behaved to guarantee nonperiodicity? I welcome arguments for or against.