Fall 2000 Exam Question 8

Hello everyone. In this video, we're going to tackle Fall 2000 question $8$.

Question: Let $f(x)=x^3-3x^2+ax+b$ be a polynomial in which the coefficients of $a$ and $b$ are real. If $-1+\sqrt{3}i$ is a root of $f(x)$, determine the values of $a$ and $b$ and find all roots of $f(x)$.

Solution

Okay, actually this has been my favorite problem on the exam thus far, which is kind of cool. So here we have a complex root, and because the coefficients are real, we know that by CJRT, we have the complex conjugate of this, $-1-\sqrt{3}i$ must also be a root, and so we know two factors.

So again, by CJRT and the Factor Theorem, we know what two factors are. So if we multiply these two factors together what do we get? So by doing a little bit arithmetic, we know that $x^2+2x+4$ must be a factor of $f(x)$.

If it must be a factor, then if I do the long division, my remainder must be $0$. So let's do the long division.

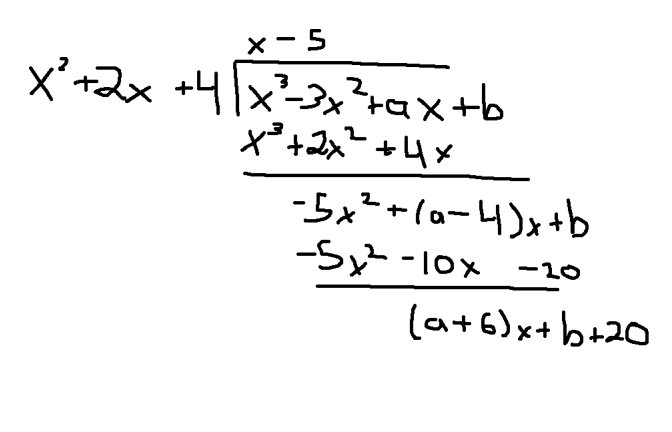
$x^2+2x+4$ into $x^3-3x^2+ax+b$. Multiply the first polynomial by $x$, I get $x^3+2x^2+4x$, simplify, I get $-3-2=-5$. $a-4$, that's $a-4$, and I bring the $b$ down.

So the leading coefficient to cancel that out I need to multiply by $-5$, so I do that. Now what do I get? I get $(a+6)x+b+20$ as a remainder.

This remainder suspiciously doesn't look like $0$, but it must be $0$, so therefore the $a$ and $b$ values must be chosen so that this polynomial is $0$.

Hey, that's easy to do. So $(a+6)x+b+20=0$, let's compare coefficients. The coefficient of x is, on both sides, should be $0$ because the right side is $0$, so that means that $a+6=0$, that is $a=-6$.

Thus $b+20=0$, that is $b=-20$, and that's it.

Very quick question, very cool. I like it, I think it's a neat little question. Very quick to solve, and that's basically it.

So what was the key? The key point here was using CJRT. So once you know one root, you know a lot of information about this polynomial. Once you know one root you know the other root, and once you have two roots, it shouldn't be too much work to find the third root.

In this case, I didn't actually find the third root I just divided and used a nice little long division trick, so I used DAP, I guess, Division Algorithm for Polynomials, but yeah I think this is pretty cool problem. This is, like I said, one of my favourite problems in this exam. Nice, short, quick solution, but it might not be the first thing you come up with. So thank you and good luck.