Abstract:

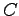
Consider a degree five curve of the form $y^2=f(x)$ where $f(x)\in \mathbb{Q} [x]$. Ulas previously showed the existence of an infinite family of curves $C$ which contain an arithmetic progression (AP) of length 11. The author also found an example of said curve which contains 12 points in AP. In this paper, we construct an infinite family of curves with an AP of length 12.