Journal of Integer Sequences, Vol. 11 (2008), Article 08.5.8

A Note on the Hankel Transform of the Central Binomial Coefficients


Mario Garcia Armas
Facultad de Matemática y Computación
Universidad de La Habana
Havana, Cuba

B. A. Sethuraman
Dept. of Mathematics
California State University, Northridge
Northridge, CA 91330
USA

Abstract:

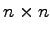
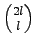
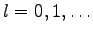
We show that the $n\times n$ Hankel matrix formed from the successive even central binomial coefficients ${2l \choose l}$, $l=0, 1,\dots$ arises naturally when considering the trace form in the number ring of the maximal real subfield of suitable cyclotomic fields. By considering the trace form in two different integral bases of the number ring we get a factorization of this matrix which immediately yields the well-known zeroth and first Hankel transforms of the sequence.

Full version:  pdf,    dvi,    ps,    latex    

(Concerned with sequence A055878.)

Received November 10 2008; revised version received November 25 2008. Published in Journal of Integer Sequences, December 14 2008.

Return to Journal of Integer Sequences home page