Journal of Integer Sequences, Vol. 11 (2008), Article 08.3.4

A Combinatorial Interpretation for an Identity of Barrucand


David Callan
Department of Statistics
University of Wisconsin-Madison
1300 University Ave.
Madison, WI 53706-1532
USA

Abstract:

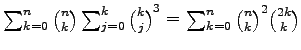The binomial coefficient identity, $ \sum_{k=0}^{n}\binom{n}{k}\sum_{j=0}^{k}\binom{k}{j}^{3}= \sum_{k=0}^{n}\binom{n}{k}^{2}\binom{2k}{k}$, appeared as Problem 75-4 in Siam Review in 1975. The published solution equated constant terms in a suitable polynomial identity. Here we give a combinatorial interpretation in terms of card deals.

Full version:  pdf,    dvi,    ps,    latex    

(Concerned with sequences A000172 A002893 A026375 and A059066.)

Received December 28 2007; revised version received August 4 2008. Published in Journal of Integer Sequences, August 4 2008.

Return to Journal of Integer Sequences home page